13 Magnetic Fields in Marine Biology

 

Learning Outcomes

• Define magnetic fields and magnetic flux;
• Explain the relationship between electric currents and magnetic fields;
• Describe the motion of charged particles in magnetic fields;
• Explain how electric fields are used by marine organisms for navigation and hunting (e.g., electric fish);
• Describe the effects of Earth’s magnetic field on marine navigation;

The Role of Electric Fields in Marine Organisms

The Earth's magnetic field plays a pivotal role in the navigation and survival of numerous marine organisms. Many species, from sea turtles to sharks, have evolved the ability to detect and utilize this geomagnetic field to navigate vast oceanic distances, helping them locate feeding grounds, breeding sites, and migration routes. These animals are equipped with specialized cells or receptors that allow them to sense the magnetic field, functioning much like a biological compass.

One of the most well-known examples is the migratory behavior of sea turtles. These reptiles can detect the Earth's magnetic field and use it to navigate across entire ocean basins, returning to the beaches where they were born to nest. Sharks, similarly, rely on the geomagnetic field to orient themselves during long migrations. They can even detect local magnetic anomalies caused by underwater geological features, which may guide them to specific locations.

Sharks and rays are particularly sensitive to magnetic fields in combination with their electroreception abilities. By sensing the electric fields produced by the muscles and nervous systems of potential prey, these predators can hunt in murky waters where visibility is limited. The ability to detect both electric and magnetic fields enhances their capacity to locate prey, even if it is buried or hidden.

Overall, the Earth's magnetic field serves not only as a navigational tool but also as a critical element in hunting strategies for species that rely on electroreception. Understanding how marine organisms interact with the magnetic field is vital for their conservation, especially in the face of increasing human-induced electromagnetic pollution and climate change.

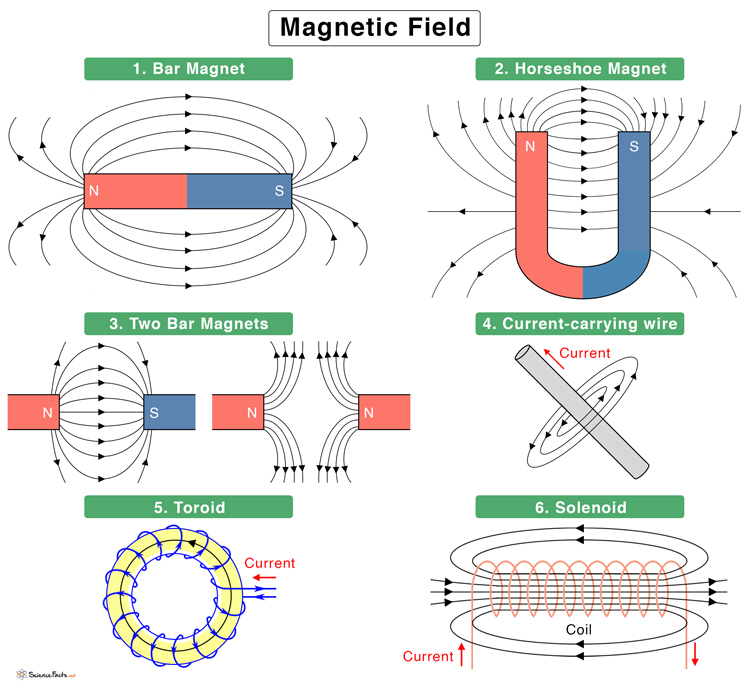
Magnetic Fields and Magnetic Flux: Definitions

A magnetic field is a region of space where magnetic forces are exerted on moving electric charges, such as electrons. It is generated by moving electric charges (currents) or by certain materials like magnets. Magnetic fields are represented by lines of force that point from the north pole to the south pole of a magnet, and their strength and direction at any given point are indicated by the magnetic field vector. The basic unit of a magnetic field is the Tesla (T), or alternatively Gauss (G), where 1 Tesla = 10,000 Gauss.

Magnetic fields play a crucial role in many physical processes and biological systems. In the context of marine biology, Earth's geomagnetic field is essential for the navigation abilities of many animals, such as sea turtles, sharks, and migratory fish, which can sense the magnetic field to orient themselves and navigate long distances.

Magnetic flux refers to the total quantity of magnetic field passing through a given area. It is a measure of how much magnetic field "flows" through a surface, and it depends on both the strength of the magnetic field and the size of the area through which the field lines pass. Mathematically, magnetic flux (Φ) is defined as the product of the magnetic field strength (B) and the area (A) through which it passes, adjusted for the angle (θ) between the field lines and the surface normal:

        Φ = B ⋅ A ⋅ cos (θ)

Where:

• Φ = magnetic flux (in Weber, Wb)
• 𝐵 = magnetic field strength (in Tesla)
• 𝐴 = area through which the field lines pass (in square meters)
• 𝜃 = angle between the magnetic field lines and the normal to the surface

In marine biology, magnetic flux can be thought of in relation to how animals, like sharks or sea turtles, detect variations in Earth's magnetic field strength as they navigate across the ocean. These animals are able to sense magnetic anomalies or local variations in magnetic flux, helping them with orientation and long-distance migration.

I. Problem Set: Magnetic Flux

1. A sea turtle is migrating across the ocean. The Earth's magnetic field has a strength of 𝐵 = 0.00003 T (30 microteslas). The surface area of the turtle's specialized magnetic receptors is 𝐴 = 0.01 m². If the magnetic field lines are perpendicular to the receptor surface, calculate the magnetic flux through the receptors. 
2. The magnetic field at the surface of the ocean has a strength of 𝐵 = 0.00005 T. The area of the surface is 𝐴= 100 m², and the magnetic field is oriented at an angle of  𝜃 = 90⁰  to the surface. Calculate the magnetic flux through the ocean surface.
3. A migratory fish uses Earth’s magnetic field to navigate. The magnetic field strength is 𝐵 = 0.00004 T, and the area of the fish's sensory organs is 𝐴 = 0.02m². If the magnetic field lines are at a 60⁰ angle to the organs, calculate the magnetic flux.
4. A ray uses the Earth’s magnetic field to detect its orientation. The magnetic field strength in its environment is 𝐵 = 0.0001T. The area of the ray's magnetoreceptive organ is 𝐴 = 0.02m². If the magnetic field is parallel to the organ (i.e., 𝜃 = 0⁰), calculate the magnetic flux.
5. An electric fish generates an electric field in its environment. The magnetic field strength in the area is 𝐵 = 0.1T. The area of the fish’s electroreceptor organ is 𝐴 = 0.003 m². If the magnetic field is inclined at 𝜃 =30⁰ to the normal, calculate the magnetic flux through the organ.

II. Problem Set: Magnetic Flux (Retake)

1. A marine biologist is studying the effect of the Earth's magnetic field on fish migration. The biologist places a 0.5 m² metal plate perpendicular to the magnetic field lines at an angle of 30° to the horizontal. The strength of the Earth's magnetic field in the study area is 50 μT (microtesla).
2. Sea turtles use the Earth's magnetic field to navigate across vast ocean distances. A research team places a magnetic sensor with an area of 0.2 m² on a sea turtle's shell, oriented at an angle of 30° to the magnetic field lines. The strength of the Earth's magnetic field in the region is measured to be 50 μT. What is the magnetic flux through the sensor?
3. Pacific salmon use the Earth's magnetic field for orientation during their long migrations. In a study, the magnetic field strength at a location is found to be 60 μT. A salmon has a sensor of area 0.5 m² attached to its body, and the sensor is placed at an angle of 45° relative to the magnetic field. Calculate the magnetic flux through the sensor.
4. Sharks are known to detect magnetic fields for navigation and prey detection. A shark in a study has a sensor with an area of 0.3 m², placed at an angle of 60° to the magnetic field. The magnetic field strength in the area is 80 μT. What is the magnetic flux through the sensor?
5. Manta rays may use the Earth's magnetic field for spatial orientation during migration. In a study, the magnetic field strength is 100 μT. A manta ray has a magnetic sensor with an area of 0.8 m². The sensor is oriented at an angle of 25° to the magnetic field. Calculate the magnetic flux through the sensor.

Electric Currents and Magnetic Fields: The Relationship

The relationship between electric currents and magnetic fields is a fundamental concept in electromagnetism, described by Ampère's Law and the Biot-Savart Law. In simple terms, electric currents produce magnetic fields, and this relationship is the foundation of many physical phenomena, including the workings of motors, generators, and the behavior of certain marine organisms.

When an electric current (which is the flow of electric charge, typically electrons) flows through a conductor, it creates a magnetic field around the conductor. This magnetic field can be visualized using magnetic field lines, which form concentric circles around the current-carrying wire. The direction of the magnetic field is determined by the right-hand rule, which states that if you wrap your right hand around the wire with your thumb pointing in the direction of current flow, your fingers will curl in the direction of the magnetic field.

Ampère's Law is a mathematical expression of this relationship. It states that the magnetic field (B) around a conductor is proportional to the current (I) flowing through the conductor and inversely proportional to the distance from the conductor. In its simplest form, Ampère’s Law is:

Where:

• 𝐵 = magnetic field strength (in Tesla)
• 𝜇₀  = permeability of free space (a constant)
• 𝐼 = electric current (in Amperes)
• 𝑟 = distance from the current-carrying wire (in meters) 

This law tells us that the magnetic field is directly related to the amount of current flowing through a wire. A stronger current produces a stronger magnetic field, and the field is strongest near the wire.

The relationship between electric currents and magnetic fields is central to many biological processes in the ocean. From detecting prey through weak electrical fields to navigating vast distances using Earth’s magnetic field, this interplay between electricity and magnetism is crucial for the survival of certain marine species. The ability to detect and respond to electric and magnetic fields is not only an adaptation for hunting and orientation but also an example of how organisms have evolved to exploit the fundamental principles of physics in their natural environment.